As just reported by Nature Physics, our collaboration between Hall Labs (Amherst College, USA) and my Quantum Computing and Devices (QCD) Labs has succeeded in tying the first knot solitons in quantum matter. We created these quantum knots in the field describing a dilute Bose-Einstein condensate (BEC), that is, a gas a atoms forming a superfluid.
In contrast to knotted ropes, our quantum knots exist in a field that assumes a certain direction at every point of space. Thus this so-called order parameter field is a vector field, as is a typical magnetic field and the velocity field of wind or water currents. In fact, knots have been observed before in water and light fields, but we were the first ones to take them to the quantum world in practice.
Let us first see how the knot looks like in theory:
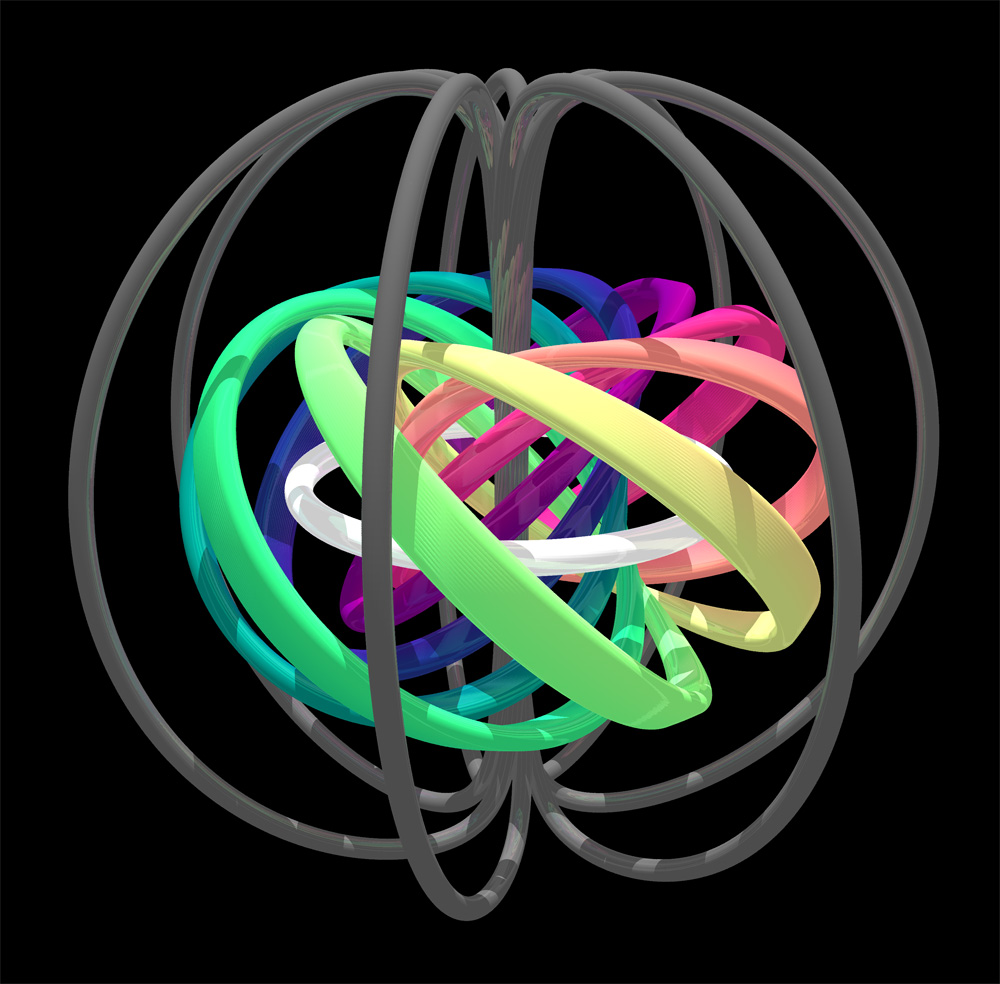
Topological structure of a quantum-mechanical knot soliton. The white ring is the core of the soliton (field pointing down), and the surrounding colored bands (field pointing sideways) define a set of nested tori that illustrate the linked structure of its field lines. The boundary of the knot lies near the dark grey lines (field pointing up). Credit: David Hall.
In the above image, you see 16 rings. Along each ring, the order parameter field is essentially pointing to a single fixed direction. However, the direction changes from a ring to ring. Thus these rings are a bit like the contour lines of constant altitude in a typical map, but more complicated.
Each ring goes through every other ring once, that is, all rings linked. Thus the resulting structure is topologically stable and it cannot be separated without breaking the rings. In other words, one cannot untie the knot within the superfluid unless one destroys the state of the quantum matter.
Now you are ready to watch our video. We first focus on the field directions that point left and right. This gives an image two linked rings (green and purple in the above image). It is amazing how well visible these rings are in the experimental images. Enjoy:
Each time an image of the condensate is taken, it is first let to expand and the different magnetic sublevels of the atoms are separated spatially. Thus we needed to make a new condensate for every frame of the video. I would had never guessed about four years ago when we started our collaboration that some day the experimental apparatus is so stable and the experiment so reproducible that we are able to conveniently make videos of the creation processes. Wow! Prof. David Hall has really tuned his BEC machine to unimaginable performance.
In practice, we tied the knot by squeezing the structure into the condensate from its outskirts. This required us to initialize the quantum field to point in a particular direction, after which we suddenly changed the applied magnetic field to bring an isolated null point, at which the magnetic field vanishes, into the center of the cloud. Then we just waited for less than a millisecond for the magnetic field to do its trick and tie the knot as show in the above video.
Previously, we have made monopoles (see also here) in a rather similar way except that we were moving the null point slowly. The technical improvement enabling us to tie the knots was that we sped up the ramp by orders of magnitude. An image of the experimental set up is shown in my previous blog post.
Here is an artistic image of our quantum knot. It looks great, for example, as a background image of your mobile phone.
Knots have been used and appreciated by human civilizations for thousands of years. For example, they have enabled great seafaring expeditions and inspired intricate designs and patterns. The ancient Inca civilization used a system of knots known as quipu to store information. In modern times, knots have been thought to play important roles in the quantum-mechanical foundations of nature, although they have thus far remained unseen in quantum dynamics.
In everyday life, knots are typically tied on ropes or strings with two ends. However, these kinds of knots are not what mathematicians call topologically stable since they can be untied without cutting the rope. In stable knots, the ends of the ropes are glued together. Such knots can be relocated within the rope but cannot be untied without scissors.
Mathematically speaking, the quantum knot we created realizes a mapping referred to as Hopf fibration that was discovered by Heinz Hopf in 1931. The Hopf fibration is still widely studied in physics and mathematics. Now it has been experimentally demonstrated for the first time in quantum matter.
Our results are the beginning of the story of realizing quantum knots. It would be great to see even more sophisticated quantum knots to appear such as those with knotted cores. Also it would be important to create these knots in conditions where the state of the quantum matter would be inherently stable. Such system would allow for detailed studies of the stability of the knot itself.


Hi Mikko,
Congratulation to your paper,is fantastic result.
I ve tried to mail you, not sure if you have recieved at all.
I do simulations on harmonic oscillators based on Fibre bundle and also lattice simulations for BEC and quantum gravity anomalies.
you might be interested to look at my results
Quantum gravity simulation
https://www.youtube.com/watch?v=lF6GqQ5QrJE
Long Range order in BEC
https://www.youtube.com/watch?v=YCgUMQ8qLq8
Self organisation in BEC
https://www.youtube.com/watch?v=RDXpHj8ArNs
the Soliton ring based on the fibre bundle here
https://www.youtube.com/watch?v=6OwSh01Eu7A
thanks
Joseph
Pingback: Here is another blog post about your work
Pingback: VIDEO: Scientists Tie Quantum Materials into Infinite Knots | »XoZZeN«
Very interesting work!